|
In crystallography, the rotation function involves a comparison of two different functions in 3-space (e.g. x,y,z) as a function of their relative orientation. The functions being compared are typically Patterson functions (although the underlying calculations may also be performed in reciprocal space). In three-dimensions, the space of possible rotations is parameterized by three angles, typically Eulerian angles alpha, beta, gamma, which range from 0 to 360, 0 to 180, and 0 to 360, respectively, for a full coverage of rotational space. However, in most crystallographic applications of the rotation function, one or both of the functions being compared have internal rotational symmetry. For example, the Patterson relating to a crystal in space group P212121 will have 222 (or D2) rotational symmetry (in addition to inversion symmetry, which is not important in the present discussions). In the presence of rotational symmetry, if a rotation function is calculated over the full space of possible rotations, then redundancy is present. For example, if one of the Patterson functions being compared obeys 222 symmetry (which implies four equivalent orientations), then there must be four points in rotation space (i.e. four different values of the Eulerian angles), for which the rotation function has identical values. More generally, if one Patterson function has rotational symmetry of order n, and the other has rotational symmetry or order m, then there must be a redundancy in the space of the rotation function of order n times m. In other words, only a fraction of the rotation function space (1/nm) is unique, and only that unique region needs to be examined when performing a rotation function comparing the two input functions. Examining a region of rotation space larger than the unique region can be more costly in terms of computation time, and can also lead to confusion because of the redundancy. The issues of redundancy and unique regions of rotation space were addressed very early on. In 1966, Tollin, Main, and Rossmann provided an analysis of the problem for situations where the Patterson functions had relatively simple symmetries, such as cyclic (e.g. P6) and dihedral (e.g. P422) (Acta Cryst. 20, 404-407). In these cyclic and dihedral systems, it was possible to specify the unique region of the rotation space in terms of simple restrictions on the values of the Eulerian angles. For example, alpha only needed to be sampled from 0 to 60 degrees instead of 0 to 360 degrees if one of the Pattersons had 6-fold rotational symmetry. However, it was noted at that time that no solution was evident for cases where the Patterson functions were of higher symmetry, namely cubic. There was no way to specify proper boundaries in terms of restricted ranges on the Eulerian angles. One could arrive at a partial solution to the cubic situations by taking account of some lower symmetry (e.g. accounting for 422 [order 8] within cubic 432 [order 24)), but this left a redundancy of a factor of 3. A few years later in 1970, the Russian crystallographer V. I. Burdina showed that a more complicated choice of Eulerian angle restrictions could reduce the redundancy in the cubic case from 3 to about 1.6, but the partial solution offered was incomplete and could not be further improved or generalized. Impetus for solving this rather fundamental problem in computation geometry may have been minimized by the development of the fast rotation function by Crowther around 1972. The fast rotation function could compare the two Patterson functions over all rotation space rapidly enough that at least the CPU costs of performing a redundant calculation were not a serious issue. Although some finer points relating to the general problem of defining the asymmetric unit of rotation space were added in the years that followed, the problem remained unsolved for some 20 years. A simple and complete solution to this problem was discovered in 1993 (Yeates, 1993. The asymmetric regions of rotation functions between Patterson functions of arbitrarily high symmetry. Acta Cryst. A49, 138-141). Arbitrarily high symmetries were covered, including icosahedral. The details are not presented here, but the crux of the solution was to use Dirichlet domains (also known as Voronoi polyhedra) as a device for dividing rotation space into unique regions. Based on this framework, it turned out that while the unique regions of rotation space could not be expressed easily in terms of Eulerian angles, they could be expressed simply (as linear equations in fact) in terms of the 9 elements of the 3 by 3 rotation matrix that describes rotation space. Interestingly, the approach generalizes easily to rotation spaces of higher dimension. Perhaps because cubic symmetries are not encountered very often, current crystallographic programs have generally ignored the problem of redundancy in rotation functions calculated for cubic symmetry, rather than implement a general solution as described. Despite the apparently fundamental nature of the underlying problem and the generality and simplicity of the solution provided, this 1993 paper has been cited only 4 times in the 20 years after its publication. |
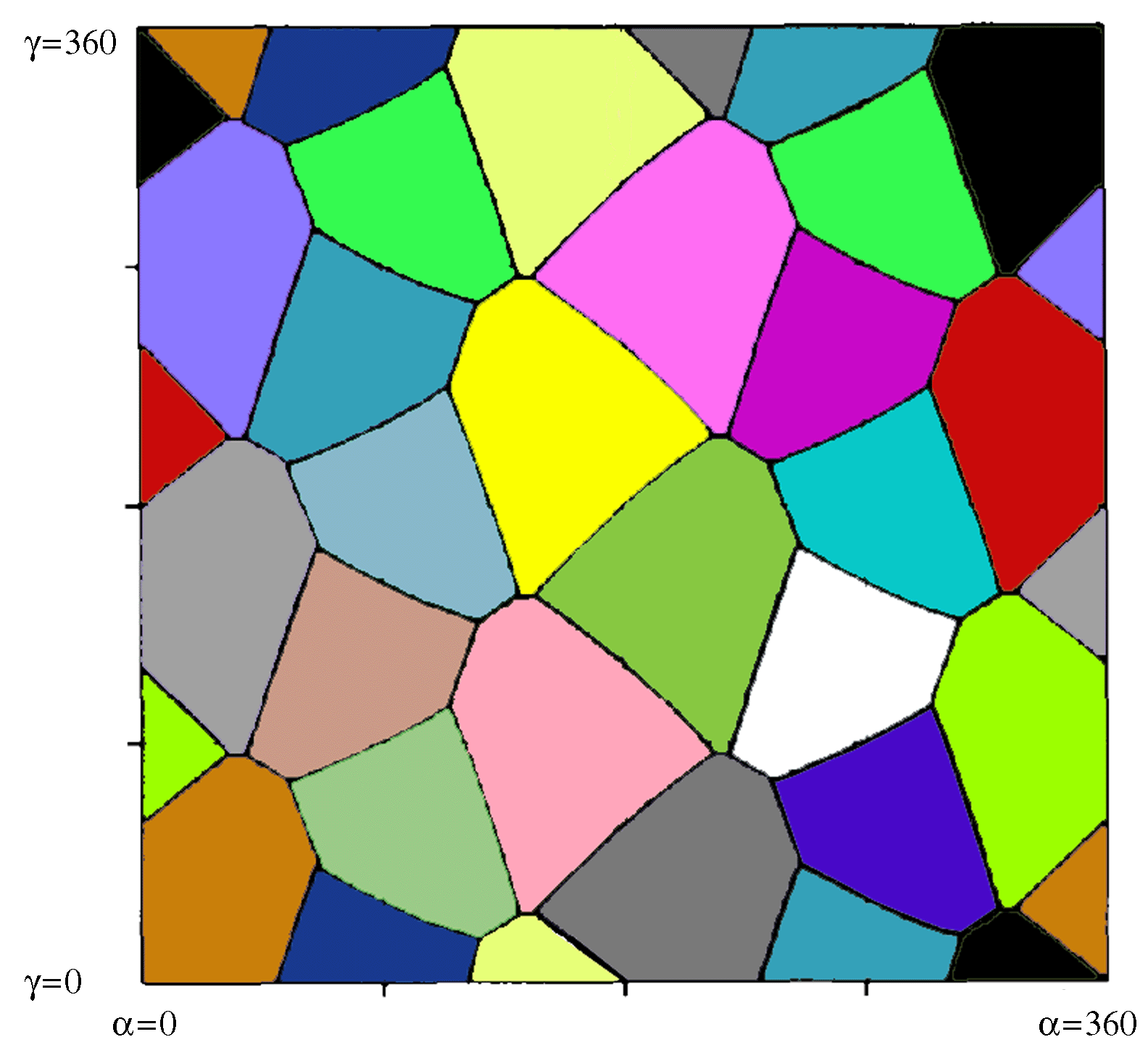 Example of rotation space divided into unique asymmetric units. The case shown applies to a rotation function between a Patterson with 3-fold rotational symmtry (e.g. P3) and a Patterson with cubic tetrahedral symmetry (e.g. P23). There are 36 asymmetric units in the full rotation space; one section (beta = 180) is shown. 24 of the 36 asymmetric units intersect this plane and are visible. |